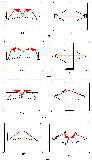Suspension

Relevant Terms:

Spring/damper: a spring and damper system with spring constant k and damping coefficient c. They will probably be provided by a mountain bike type system with either air or coil springing and oil damping.

Wheel travel: the vertical distance that the wheel moves because of suspension movement.

Spring rate: the spring constant k of the spring

Motion ratio: the ratio of wheel travel to spring/damper travel

Progressiveness: The change in motion ratio as the suspension goes through its travel.  This is reflected in a change in spring rate felt at the wheel.  A progressive suspension has “rising rate.”  The opposite is “falling rate”

A-arm: an a-shaped arm comprised of two tubes with three pivots. Two of the pivots are mounted to the frame, and the third to the upright.

Upright: the vertical member between the upper and lower a-arms that allows the wheels to be attached to the suspension system

Push Rod: a solid link between the lower a-arm and a rocker so that force from the wheel is directed towards a spring/damper - in compression
 
Rocker: a device that takes a force from one direction and re-directs it to another direction. This is usually accomplished through a pivoting or rocking mechanism.

Camber: the inward or outward tilt of the top of the wheels when viewed from the front of the buggy. The tops of the wheels facing inwards (/ \) is negative camber, wheels facing outwards    (\ /) is positive camber. Camber is measured in degrees.

Caster: when viewed from the side, caster is the tilt of the steering axis. When looking at the left front wheel of the buggy, if the top of the upright is behind the bottom of the upright, (\) there is negative caster. If it is in front, there is positive caster.  Castor is measured in degrees.

Toe in: when the front of a set of wheels point inward towards one another. Measured in degrees.

Toe out: when the front of a set of wheels point outward away from one another. Measured in degrees.

Static ride height: the height of the frame above the ground that the buggy will sit at when motionless and loaded with all drivers and accessories.

Compression travel: when the suspension compresses from static ride height and the frame gets closer to the ground

Droop travel: when the suspension extends from static ride height and the frame gets farther from the ground

Bottoming: when the suspension cannot compress any farther

Top out: when the suspension cannot extend any farther

Roll: the twist of the chassis measured in relation to the ground. The frame has 0 degrees of roll when it is standing still, but if it is cornering or on a side hill the frame will twist in relation to the ground due to the suspension compressing unevenly side to side. Measured in degrees.

Introduction / Basic design goals

The suspension was one of the most successful features of last year’s buggy.  Team members reported that our buggy ate up the obstacles in a way that gave our team a significant advantage in traversing the course.  This year’s goal was to retain the basic design of last year’s suspension but improve it in the following ways:
 

1.   Last year’s front suspension worked well, but created major problems because it forced the front axle to be moved well forward of the wheel center.  This happened because the ideal configuration for a push rod/rocker suspension design is to have the whole system in the plane defined by the arc in which the wheel center moves as the suspension goes through its travel.  If the rocker is mounted in any other plane, the push rod cannot stay in the same plane through the travel.  The effect is minor on cars, because they have relatively small travel, but is amplified on a buggy with 8 inches of wheel travel.  The rocker could still be designed out of plane, but such a design would necessitate a very sturdy rocker mounted on a very sturdy pivot, all of which would be heavy.  For all these reasons the 2001 team decided that the suspension should stay in the ideal plane.

This decision forced the roller clutch assembly to be moved forward.  The result was very high friction in the constant velocity joints that were used due to the high angular misalignment at the upright.  In addition, The fact that the axle had to go around the suspension forced last years team to use a very small gear on the roller clutch assembly where they would rather have used a large one to get correct gearing.  Even with the small gear, there was often contact between the chain ring and the suspension, causing damage to the shocks, and often derailing the chain.  This situation is one of the major problems our new design must solve.

Last year’s front suspension is the most relevant for this year’s design, because our back to back rider configuration will make both buggy halves similar to last year’s front half.  However, Last year’s rear rockers provide another perspective on the matter.  The rear suspension was actually mounted slightly out of the ideal plane to provide clearance for the seat.  This resulted in side loading of the rockers, as described above.  The suspension worked well, but during testing this year, one of the rear rocker mounts failed, presumably from the side loading it experienced.  This provided us another point of consideration in designing our new suspension.

Last year’s rear mounting scheme, though mounted out of plane, did not completely solve the roller clutch assembly clearance problem.  Therefore it is not directly applicable for solving the space constraint problem described above.

 
2. The rocker suspension concept is used primarily because it allows the motion ratio and progressiveness to be adjusted by choosing the appropriate rocker geometry.  However, despite this convenience, last year’s buggy was too soft on the front, and too progressive on both the front and the rear.  Presumably the former problem could be solved but changing to stiffer springs, but there is no solution to the progressiveness problem short of rebuilding the suspension system.  Last year’s time consuming CNC design and manufacturing process, along with the expense of the aluminum used to make the rockers made this correction difficult to achieve.  Because it is very difficult to calculate the exact progressiveness, especially for a three dimensional system, and because it is even more difficult to predict what level of progressiveness will be ideal, we feel that it should be easily adjustable.  This is a second goal for the new suspension design.

A-Arms

Design Notes:

    Geometry:
 

In designing the suspension we first decided what performance criteria we wanted it to meet.  Based on the success of last year’s suspension in handling the course, we decided to retain the same basic criteria as last year:
· 8” of suspension travel (2” sag to ride height, 6” additional travel)
· Rising rate of ~30% (this was designed for last year, but arguably not actually achieved)
· Adjustable ride height for different sized riders
· Maximized ground clearance while keeping rider weight reasonably low
· Ideal kingpin and appropriate caster angle (this was new this year).
· Minimized side-loading on wheels

Because our buggy needed to have the wheels overlap when folded, the total width at the rear wheels was designed to be 4” narrower than the front.  This allows two-inch wide tires to overlap each other in the folded position.  We decided the front wheels should be as wide as possible (for cornering stability) while still fitting in the 4’ box.  We decided the outermost point (the outside of the hubs) should be 47” just to leave a small margin for error.  Since the rear was 4” narrower, we were worried the A-arms would be so short that there would be excessive camber change through the travel.  For this reason we moved the upper A-arm attach points to the top of the frame bars, and narrowed the frame width from 10” to 9”.   For symmetry, we did the same with the upper A-arm attach points on the front.  We considered having the A-arms pivot inside the frame tubes to reduce bending moment on the tubes, but decided it was unnecessary because the upper A-arms take very little load (essentially only cornering loads).

Standard mountain bicycles use 26” wheels.  For ease in acquiring parts, we decided to use this size.  Based on the experience of those who attended the competition last year, we felt these wheels would be sufficient to handle the simulated lunar course.  This meant that the wheel axle would be constrained to be 13” above the ground. Due to the sinusoidal effects of U-joints at large angles of action and frictional considerations, we wanted the drive-train in line with the axles at ride height.  This also constrained the roller-clutch assembly to be 13” above the ground at ride height. To maximize clearance, we decided to mount the roller clutch assembly as close to the bottom of the frame as possible.  This put the top of the frame at 17.5” above the ground (at ride height). Having the top A-arm horizontal at ride height is ideal for the camber response we want our suspension to have in roll: negative camber in compression, positive in droop.  The top A-arm has the most effect on this because it is shorter.

For our upright to reach around the disc breaks on the rear, and for good clearance while keeping the push-rod attachment point low, we wanted the lower A-arm to slant down towards the wheel.  To accommodate a 6” disc break and put the drive axle roughly in the middle of the upright, we settled on 9” vertical distance from pivot to pivot on the upright.

( Sketch A-arm geometry )

By looking at last year’s buggy we initially chose the distance between the a-arm pivot points on the frame to be approximately 12”.  This would provide the proper geometry to take the horizontal forces from the wheels during acceleration, braking, and obstacle encounters.   To keep jigging and manufacturing simple, we decided to leave the A-arms symmetrical front to back.  We saw no reason this would be a problem.
 
The upside-down triangle frame design produces increased negative caster on the outside wheel of a turning buggy.  This helps to reduce the undesirable side loads.  Last year’s buggy actually had a significant amount of negative caster at ride height, presumably for this purpose.  This made sense, but such a design interfered with one of our planned improvements to last year’s design.

One of our biggest complaints about last year’s buggy is the poor steering response.  This year, we hoped to solve the problem by designing appropriate kingpin angle into our suspension to minimized bump-induced steering and keep us from going off the course due to collisions with the simulated lunar obstacles.  Kingpin angle is the angle of the upright-A-arm attach points from vertical when viewed from the front.  Proper tuning of this angle allows the turning axis to go through the point where the wheel meets the ground.  This eliminates bump induced steering and produces a light steering response.  We felt this was a key improvement that could be made to our buggy design, and wanted to incorporate it.  However, if we designed for negative caster at ride height, this would require a very large kingpin angle.  Such a large kingpin angle would result in a very short upper A-arm and therefore in excessively progressive camber.  It would also cause constant side loading of the wheels at ride height.  For all these reasons we decided to design for zero camber at ride height.  The lower upright-A-arm attach point was put as close as possible to the spokes, resulting in a kingpin angle of about 8 degrees.

The use of kingpin introduces another steering problem.  If the upright-A-arm attach points are on a vertical line when viewed from the side, then turning will cause both of the wheels to have positive caster.  This is actually desirable for the inside wheel, but very undesirable for the outside wheel because it increases side loading.  The solution to this problem is to make the upper attach point further back than the lower attach point.  This is called caster angle.  This also causes the turning axis to hit the ground slightly in front of the point where the wheel hits the ground.  This will produce a slight self-centering tendency in the steering, which is desirable.  An appropriate caster angle is one that is roughly the same as the kingpin angle.  We calculated that the upper A-arms should be attached 1.25” behind the lower ones.

        A-arm tubing size calculations:
 

Calculating the tube sizes for the A-arms turned out to be a much more difficult problem than we first anticipated. To choose the sizes we first needed to know what stresses were involved. These were determined by our loading criteria. We stated that the buggy must be able to

1.) Corner in excess of one gravitational constant
2.) Survive a two-foot fall
3.) Clear a six-inch curb traveling 20 mph

· Cornering

Last year these calculations were done by finding the centrifugal force acting on the buggy in the turn (one or one and a half times the total weight) and dividing that between the contact patches of the outside wheels. With a total buggy weight of 100 lbs empty + 350 lbs riders, each wheel would need to carry 340 lbs or so with a 1.5 safety factor. However, we were using bicycle wheels that last year’s team had calculated to fail well below 200 lbs. We decided that a 200 lb side force at the contact patch of the wheel was a reasonable upper limit including safety factor. Last year the team calculated this turn while braking (add a force vector directed against the motion equal to half the weight times the friction coefficient). We decided that for this loading condition braking was negligible since the brakes were rear mounted and would tend to break the rear end loose, putting it in kinetic friction mode. From this 200 lb side loading condition it was easy to calculate the forces on each A-arm using force couple and trigonometry. The tip of each a-arm creates a force couple to counter the torque from the contact patch. Each A-arm pushes about the axle with 195 lbs. of force. Dividing this force into the two tubes using sum of the forces to zero yields:

Forces on front upper tubes each (tension) 210 lbs
Forces on front lower tubes (compression) 200 lbs
Rear upper tubes (tension) 212 lbs
Rear lower tubes (compression) 205 lbs

These were the forces just to keep the buggy in the curve. The suspension pushrod added another vector to the a-arm node. For cornering this force actually countered the contact patch induced torque thereby reducing the forces on the A-arms.

· Two Foot Drop:

The next loading condition was a two-foot drop. Due to the suspension we estimated a 400 lb average vertical force at each wheel with the maximum at bottom out of 600 lbs. This would put the bottom A-arm in tension with 1600 lb force. Using the same methods as before yielded:

Forces on front upper tubes each (compression) 860 lbs
Forces on front lower tubes (tension) 835 lbs
Rear upper tubes (compression) 870 lbs
Rear lower tubes (tension) 840 lbs

This would be the effect of landing on ice, i.e. the wheels do not give inward force. If landing on pavement or dirt there will be a significant force inward by the wheels to counter increasing camber throughout travel. This would oppose the compressive forces on the upper A-arms.

· 6” Curb:

The final loading condition was the 6” curb. This was by far the most difficult to calculate. In the end we modeled the system using Working Model 2D. The first time around we did not account very well for the give in the tires (simply modeled it as solid rubber) and found the impact force to be enormous. After changing the model a little bit the numbers were reasonable. Instead of having a rubber wheel, we put in a spring to represent the tire. We realized that although it may be feasible to make a buggy that could withstand a 6” curb at 20mph, it was not possible to make a tire and wheel that could withstand that impact. Assuming a square profile of the curb, we estimated the k value of the tire to be around 240 lbs/in at 60psi (with the curb 1” into the 2” wide tire roughly 4 sq in are displaced: 4 x 60 = 240) and 480 lbs/in at 120psi. We assumed mild damping of around 250 N-s/m. Assuming that the tire should not compress more that 1.5” less the curb hit the rim and buckle it, we modified the loading condition to 10 mph into the curb with 60psi tires. The results from the program are below:

· Hitting a 6” Curb at 10 MPH

Wheel:
Max x-direction: 650 lbs
Max y-direction: 400 lbs

Max loads on back tube of the lower-front a-arm: 900 lbs compression,
Max loads on front tube of the lower-front a-arm: 1300 lbs tension
Max loads on back tube of the upper-front a-arm: 900 lbs compression
Max load on the front tube of the upper-front a-arm: 900 lbs tension

These were the most severe loadings so we calculated tube diameter from these values. We used Euler’s buckling formula for pinned-pinned loading conditions to find the necessary tube diameter with 0.035” wall thickness.

Pcr = pi^2EI/L^2
 

For these loading conditions the closest diameter that met the specifications was 5/8” diameter. For the front half of the A-arms 3/8” tubing would have been ok. However two things prevented us from using this. First there was the kick factor. We were afraid that running into an obstacle or hitting the tube would buckle it regardless of the wheel loading. Second, manufacturing would be made much more difficult by using unequal sized tubes. The extra weight of using 5/8” instead of 3/8” on all the front tubes was:

(0.2205 lb/foot - 0.1271 lb/foot)11ft=1.0274lb

Saving one pound with the smaller tubing was not worth the added manufacturing cost and risk factor.

· Some Thoughts:

Possibly the 6” curb loading condition should be rethought. Hitting a curb at 20mph is a fairly severe impact (a car wheel would likely buckle) and not very realistic for the racecourse. In addition, modeling the situation is needlessly difficult. Another method to determine tube loading is to attach strain gauges to the current a-arms with a voltmeter that records a maximum value. Then ride the buggy into curbs around corners and everything to get realistic numbers. Even though the calculated numbers say we are ok, there is no better method than taking experimental data. The a-arms may still be needlessly overbuilt.

Fabrication Notes:

In order to drastically improve the preciseness of the construction of this year’s buggy, we set out with a goal to use an accurate jigging and mitering process wherever possible on this year’s suspension.  One main jig was constructed in order to ensure that A-arms were fabricated to meet correct length, angle, and position requirements where they were designed to fit on the buggy.  This process enabled us to construct 8 sets of A-arms that fit the exact specifications of the design.

The first step in the construction process was to figure out a way to accurately cut the tubes to fit to the inserts. The goal here was to quickly reproduce a good enough miter to weld to.  By ensuring the A-arms were constructed as specified we were able to make certain that the suspension would perform to it’s maximum potential.  The main problem we had to solve was keeping the two miters in the same plane.

Some of the first ideas were to tilt the mill in such a way as to cut the hole to the specified angle.  At first this fell through because we could not figure out how to ensure the miters were in the same plane.  Another idea was find a way to drill an accurate hole and miter the rest of the tube using a grinder or a sander.  This idea however is not very accurate and does not lend itself to reproducing 16 cuts.  In desperation, we consulted Glen Swan as to how he cut the angles in the tubes he uses to make bike frames.  The solution was surprisingly simple.  To keep the two miters in plane, two pairs of half-moon shaped  blocks were clamped to the tube prior to mitering, one on each end.  This was done on a flat table so that the blocks were in the same plane.  We then constructed a clamping apparatus that could be attached to a lathe where the normal cutting or turning tool would attach.  This clamped onto one of the half moon blocks that were clamped to the tube.  The tool mount was then accurately set to the correct angle to ensure the hole was cut at the proper angle.  In normal lathe operation the piece spins whereas the cutting tool stays stationary.  We reversed this process and placed a 5/8” hole cutter in the chuck of the lathe while keeping the tube stationary.  The process allowed us to cut all 16 A-arm tubes with complete accuracy while using only one machine set up.  Am similar setup could have been used on the mill, but tilting the mill is slightly more tricky than adjusting the angle on the lathe.

The 8 A-arms were constructed using 5/8” 4130 steel tube with .035” wall thickness. Inserts were made to provide a place for the rod ends to be screwed in.  All inserts were made out of solid steel stock and turned down to 0.555” outer diameter.  The inserts were then cut to a length of 1” and drilled and tapped to fit the accompanying rod-ends.  All of the frond rod ends were 3/8-24 and the back rod ends were 5/16-24.  The front rod ends were larger because our steering required high misalignment rod ends.  The front rod ends had to be mounted with the bolt vertically to handle the steering angles, whereas the back could be mounted with the bolt horizontally.  Therefore the front rod ends had to reach large enough misalignment angles to handle the whole suspension travel.  The misalignment requirements on the rear were minimal.  We wanted no less than ¼” bolts to go through the eye of the rod ends to handle to handle the breaking, steering and suspension loads.  For high misalignment rod ends, the ball needs to be much larger for a given eye diameter.  The high misalignment series is therefore constructed larger and beefier in general.  This is why the thread on the front was larger diameter than that on the rear.  In the end it makes sense, since the front will encounter obstacles while the buggy is still at speed, and will therefore experience higher loads.

The next step was to construct a jig that lined up the tubes around the insert at the correct angle.  We used a large flat piece of aluminum with numerous attachment points in order to accommodate the various lengths and widths of the A-arm.  Once the lengths and widths of the A-arms were determined we were then able to set up holes on the jig plate that correctly held a mock aluminum frame in place to constrain the movement of the tubes during welding.  A block was attached to one of the plate with a bolt placed threw it to hold the insert in place.  This held the two bushing inserts in the correct location and the tubes were then fitted around these inserts.  These inserts were 5/8” OD tube that were later fitted with brass bushings in order to allow the A-arm to travel smoothly over the bolt.  Now the A-arm tubes were completely constrained in all directions to the exact location of the design specifications and were ready to weld.  Tabs were the welded to the frame at a distance equal to that of the insert fitted with the brass bushings.  By jigging up all the parts for the A-arms were able to ensure that they were all constructed to meet the exact specifications required in the design. 

Performance / Lessons Learned:

Overall the performance of the A-Arms lived up to all expectations.  The Caster and Kingpin angles ended up being correct and they were both a huge success.  This year we used thinner A-arms without any adverse effects and they seemed to hold up just fine despite not being attached perfectly at the nodes.  The camber change through travel was also very good, and the wheels held up just fine.  A big change on this year’s buggy is that the A-arms are interchangeable and could easily be replaced if broken.  This is much better than the previous design, which required side specific arms that could only fit in one place.  The addition of hex bolts at all frame connections helped save time in assembly and added to the ease of interchangeability of the A-Arms.  We decided to use ¼” bolts at upright-A arm attachment points because they were readily available, not because of calculations.  These bolts might be too small, but seem to have held up fine through all riding to this point.

Rocker Assembly Introduction

We discussed several options for solving the roller-clutch assembly clearance issue described in the introduction .  The first option was to leave the suspension design exactly the same and try to solve the problems by modifying other systems on the buggy.  We had already decided to use universal joints rather than constant velocity joints on the outboard ends of the half shafts to save weight and reduce friction.  This would solve the high-angle friction problem we had last year.

The chain ring clearance problem however was still present, and was actually exacerbated by our back-to-back rider configuration.  For the rear rider to pedal forwards but go backwards we had to incorporate a gear reversal mechanism.  For various reasons, we decided to wrap the chain around some guides in front of the rear roller-clutch chain ring.  This made it all but impossible to build the rear drive-train around the suspension.  We also made the decision to mount the steering linkages inside the frame rather than below it.  This made the space tighter in the front half of the buggy as well.  We decided that the suspension would have to be put somewhere else.

There were three major options we considered for relocating the suspension.
1. Keep the suspension in the correct plane, but widen the attach points of the rockers until the shocks are nearly horizontal.
2. Rotate the plane of the rockers towards the middle of the buggy around vertical axes through the wheel centers.
3. Mount the suspension in planes defined roughly by the top outside frame members and the point of the lower A-arms.        

       
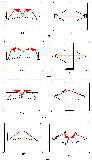
Options considered for rocker-pushrod arangement

The first option solved the clearance issue, but had two major problems.   The first was mounting the rockers to the frame.  Making the shocks nearly horizontal meant that the mounting points needed to be at least 18” apart.  This could have been achieved by building extensions on the frame, but they would have had to be very strong to take the high loads from the wheels.  This could have been done, but would have involved extra work and extra weight.  The second problem was more serious.  We had decided that the one-inch tubing used for the lower A-arms last year was excessive for any loading conditions with the exception of the bending moment caused by the push rods.  We wanted to decrease this tube to 5/8” to reduce weight.  This required attaching the push rod as close to the node as possible.  To do this, the push rod could not come straight down to the attachment point, as it did in last year’s suspension, and in option 1.  This would have caused interference with the half shaft, particularly on the front where the steering causes the half shaft to move from side to side on the outboard end.  The solution we decided on was to have the push rod to come down from the back to clear the half shaft, attach to the node of the A-arm, and be properly positioned to encounter bumps hitting the front of the wheel.

Option 2 solved the problem mentioned above, and made clearance for the roller clutch assembly.  Several problems with this design however forced us to abandon it as well.  Keeping the rockers vertical and moving them towards the seat created potential interference with the rider’s legs.  We had planned to keep the rider’s rear as close to the top of the frame as possible to keep the center of mass low for cornering.  We feared that Option 2 would prevent us from doing that, or else cause discomfort or even prevent the rider from pedaling.  In addition, the placement of the shocks interfered with our intention to move the steering inside the frame.  This design was also susceptible to the problem of side loading the rockers we discussed earlier.

Option 3 was the ideal choice as far as interference was concerned.  With the shocks mounted horizontally and lengthwise on the top of the frame we could eliminate all interference issues with the drivetrain, the rider and the steering.   This solution also eliminated interference with the half shafts and properly supported loads due to hitting bumps with the front of the wheels.  For these reasons we decided to try to solve all the problems associated with option three and implement it if possible.  There were four major problems we had to solve:

1. The geometry was out of plane, and could potentially have lead to severe side loading of the rockers.
2. Mounting the rockers on top of the top frame bars would put the frame members in severe bending and torsion.  The frame would not withstand these forces without a lot of reinforcement.
3. The rockers and shocks would have to be kept far enough apart not to hit each other, and to leave enough clearance for the chain to pass from the hub shifter to the roller clutch assembly.  This meant some support structure would have to be built outboard of the frame.
4. This design put the push-rods at a smaller angle with the horizontal than the other designs.  This meant that the push-rods, rockers and shocks would have to withstand a larger total load to support the same vertical load at the wheel.

The first problem was solved by allowing the rockers complete rotational freedom around their attachment points.  They were mounted with a high-misalignment spherical bearing capable of traveling 23 degrees in each direction.  The rocker was then mounted to both the shock and the push rod using cylindrical pivots, to keep those three elements always in plane.  The plane is defined by the rocker-frame attachment point, the shock-frame attachment point, and the varying attachment point between the push rod and the A-arm, each mounted with a spherical bearing or rod end.  This set up allowed the rocker to adjust to varying push rod angles, and thereby eliminate any side loading of the rocker.

The second problem had to be solved in a way that was strong enough but not too heavy.  The solution to problem 2 would also have to solve problem 3.  We decided the best method was to put a horizontal beam under the top rails of the frame to support the rockers.  This could be extended out beyond the width of the frame to solve problem 3.  This also helped to lessen the difficulties we faced with problem 4 by increasing the angle with the horizontal.  The horizontal beam would be slightly thicker and wider than the stock we used on the rest of the buggy.  We calculated that 1” x 0.058’ wall would be strong enough to support the largest loads we expected out of the suspension.  The tabs that would support the rockers were made out of 0.063” sheet, and reinforced to prevent buckling.  Though this was some of the heavier duty construction on the frame, it would contribute significantly to it’s weight, but we decided this was appropriate given the that the entire weight of the buggy is supported through the suspension.  This design did not account for any lateral loads this cross member might have needed to support, but we intended to attach the trussing directly to the outermost part of the cross member, so it would help support any side loading and transfer the weight of the buggy to the suspension.  The crossbar had an additional benefit of providing a potential mounting point for the roller clutch assembly, which would have to be arranged for somehow anyway.

Problem 4 simply required evaluating the weight impact that would result from making the suspension strong enough to support the loads involved.  Calculations revealed that 5/8” x 0.035” tubing at 16” long would be strong enough to take 1000 pounds in buckling (with a safety factor of 2).  This was the maximum predicted loading of our suspension, given critical damping and impacting a curb 6” high at 10 mph.  The length of the push rods in our final design turned out to be somewhat less, roughly 12”, so we were confident that this would be sufficient.  The rockers would be made out of much thicker material than would have been necessary to withstand buckling, the reason being to prevent pullout of the pins at the attach points.  We were therefore not concerned with their ability to handle the compressive loads.  (Rocker design will be addressed more completely in the rocker section)  Making the shocks strong enough simply involved choosing an appropriate spring rate.  (More on this in the shocks section)  Therefore, the only impact on weight due to the increased load on the suspension was in the push rods and the crossbar on the frame.  This amounted to less than a pound on the whole buggy, so we were willing to take the additional weight for the clearance benefits.  In the end we settled on option 3.

Shocks

Design Notes:

Last year we used Fox’s coil spring shock line, the “Vanillas.”  The reliability, durability, adjustability, and low cost of these shocks made them a perfect choice for the old buggy. Fox shocks are not the most inexpensive shocks available, however their many features make them ideal for our use.  Fox shocks are available at any bike shop and have many different strength springs available. They also have externally adjustable compression and rebound dampening knobs, allowing them to be tuned for the buggies suspension easily and on the fly.

                                                             
         Vanilla Shocks                                                      Float Shocks

We considered using Fox’s air shock line, called the “Floats” this year.  The advantage to these is that they offer adjustable spring rate, which would allow us to quickly adjust for different riders.  This is not a necessary feature for the competition, since all of the riders we are considering are roughly the same weight.  It would allow us some flexibility in calculating the required spring rate (which is no easy task, given the complexity of the suspension, and our uncertainty about the buggy weight, the exact distribution of rider weight and the exact magnitude of the forces we are likely to encounter).  If we bought springs and found they were the wrong spring rate, it would cost us $50 to replace them.  The floats do not offer quite as much travel as the vanillas, but this would not be a problem since we could adjust the rocker geometry as necessary to compensate.  The main disadvantage to the floats is that they require more maintenance and are less reliable.  They must be checked before each ride, since the air can leak out around the seals.  They are also less durable and therefore more prone to failure.  In the end, partially for reliability, and partially due to the success of last year’s suspension, we went with the vanillas.

Because of our high travel requirements, we used the highest travel Vanilla’s available.  These are 8.75” long with 2.75” of travel. Springs are available with spring rates from 300 lb/in to 1200 lb/in in 50 lb. increments.  There was some confusion in calculating the necessary spring rate, because loading calculations for the front included the damping loads the springs would have to handle.  Due to a miscommunication, these same loading values were used to determine the necessary spring rates, and the shocks we purchased were too stiff.  Since accurate calculations were never done on the rear, we had to guess what spring rate was necessary there.  In the end, after some mixing and matching with last year’s buggy (which was too soft on the front anyhow) we achieved spring rates that felt right on the buggy.  We used 450 lb/in springs on the front and 300 lb/in on the rear.  The smaller spring rate on the rear was due partially to the fact that the rear rider is much lighter than the front, but was also due partially to the narrower wheels on the rear which resulted in a steeper push rod angle, causing the push rod to take less load for the same wheel loading.

Rockers
 
The rockers on the 2001 buggy were CNC’d out of aluminum.  This year’s team lacked the necessary experience to do this.  We were forced to seek alternative designs.  For the same reasons we chose it for other parts of the buggy, we leaned towards chro-moly for its manufacturability, low cost, strength and weldability.  The idea was to use two parallel plates with holes drilled in them for pins.  Theoretically this could have sufficed for the entire design, however, the fact that the rocker and pins would have to keep the entire linkage system in plane made two floating plates undesirable because they could rotate relative to each other, allowing the pins to twist out of the correct plane.  A box section on the compression side was desirable because it would prevent the two plates from buckling.  This however would not be completely sufficient to keep the two plates from twisting relative to each other.  Another factor came into play as we tried to solve this problem.

Since we had decided to go with option 3, the rocker-frame attachment point had to house a spherical bearing.  The question was whether to mount this bearing to the rocker or the frame.  The spherical bearing would have to be pressed into a precisely machined tube attached to either the frame or rocker.  One of our goals was to make our rockers quick and easy to produce, so putting the bearing in the rocker could have made this difficult.  On the other hand, putting the whole frame in the arbor press could have been a daunting task.  Also, if the tube were mounted in the rocker, it could provide it with additional torsional strength.  That, in conjunction with the stiffening member on the compression side, would keep the plates from twisting relative to each other.  With some further thought we realized that we could turn a substantial length of tube down all at once, and cut it into many spare bearing-tubes for making extra rockers.   Therefore, we decided to mount the bearings in the rockers.  It is worthwhile to note that we came up with no strong arguments either way on this point, and it could easily have been done either way.

The final design decision to be made about the rockers was how far apart the two plates should be.  Since the rocker was going to pivot between two parallel plates attached to the frame that would support the pin through the spherical bearing, it would need to be as narrow as possible.  The width was ultimately decided by how wide the shock was at the attach point after the bushings were installed.  The rocker plates were 5/8” apart, ¾” wide on the outside.

Design Notes:
 
    Rocker Geometry:

When designing the rocker geometry, we had two main concerns:

1. The motion ratio (wheel travel/shock travel) had to be appropriate to get the 8” of wheel travel we wanted from the 2.75” of travel in our shock.
2. We wanted a rising rate to allow the suspension to handle small bumps well, without bottoming out under heavy loads.

Once these goals were accomplished, we could tune ride height and maximum loading by adjusting spring rate and preload on the shocks.  Damping and rebound are also adjustable on the shocks.

We decided that since our push-rod-rocker-shock assembly was in plane, a two-dimensional computer-modeling program would be ideal to tune our rocker geometry.  The problem turned out to be more complicated than this.  The out-of-plane design of our suspension caused the rocker moves in a complicated plane that changes throughout the suspension travel.  The A-arms on the other hand, moved in a vertical plane.  It turns out that the motion ratio and progressiveness of the suspension is affected by the geometry in both planes.  The motion of the push-rod and A-arms in the vertical plane creates a large rising rate which is built into the design.  This rate was so large that we had to counteract it by designing a large falling rate into our rockers.  The problem was so complicated that it was hopeless to solve it without the aid of computer modeling.  However, the only software that was available to us was working model 2-d.  This software allows rods, ropes pulleys, springs and dampers to be modeled in a two-dimensional environment.  (Apparently a 3-d version of this software exists, but we had neither the resources or the expertise to use it)  In the end, we made a complicated model that connected the motions in the two different planes.  With a number of approximations, we felt we had created a model that approximated the motion of the buggy reasonably well.  Using this model, we tuned the rocker geometry to give us the performance we wanted.

The motion ratio was controlled by changing the ratio of the radii of the shock attach point and the push rod attach point as measured from the frame attach point (Rs and Rp in the figure).  Greater Rp / Rs results in greater motion ratio.  The progressiveness was controlled by changing the angles theta-s and theta-p shown in the figure (these angles are defined when the suspension is at top out).  The progressiveness relies on the nonlinearity introduced by the arcs that the attach points travel through.  This nonlinearity results from the change in mechanical advantage as the angles theta-1 and theta-2 change throughout the suspension travel.  Therefore, tuning the rate also involves choosing an appropriate size for the rocker (that is, two inches of shock travel must introduce an appropriate amount of nonlinearity to produce the desired progressiveness

Using an iterative method, we eventually settled on a front rocker design that satisfied our design criteria.  Unfortunately, a corrupted floppy disk and lack of time to start the program over again prevented us from doing the same for the rear rockers.

The geometry on the rear was significantly different from the front, mostly because of the narrower wheel placement.  We used the same rockers from the front on the rear, hoping they would work, but found that they were too progressive and too stiff (the latter problem could not be solved by changing springs because we were already using the softest ones available).  We fixed these problems by modifying the attach points on the rear rockers until we were satisfied with their performance, and then constructing new ones.  In this situation, we were glad for the simple and low cost design of our rockers.

The working model 2-d software not only helped us fine tune our rocker geometry, but calculated the loads that could be expected in the push-rod and on the frame, helping us decide what size stock would be necessary at those locations.

    Pins and Bearings:
 

The most critical pin and bearing decision on the rockers was the frame-attach-point.  This point would need to support the resultant force due to both the shock and push rod.  With the shock fully compressed using 450-pound springs it would put roughly 1000 pounds of force on the rocker.  A slightly smaller force from the push rod would be oriented at roughly 90 degrees to the force from the shock (slightly smaller because the push-rod/shock motion ration was slightly greater than one).  A rough estimate of the resultant is 1500 pounds, inwards and towards the back of the buggy.  The spherical bearing would have to house a pin strong enough to support this load.  The bearing would have to have high misalignment tolerance because the suspension was designed to move the push-rod through more than 30 degrees.  This meant the OD of the bearing would have to be much larger than the pin, and too large of a pin would make the bearings very heavy.  In addition, enough space would have to be left on top and bottom of the bearing to allow the rocker its full range of motion.  Care would have to be taken to avoid putting excessive bending moments on the pin.

Calculations showed that a 3/8” pin would support the loads with a safety factor close to 2.  This corresponded to a high-misalignment spherical  bearing with an OD of 0.906” and an eye length of 13/16”.  This bearing was actually pretty difficult to track down, because National Rod Ends as out of stock.  We ended up getting them from Kaman, but they were not cheap.  At almost $40 apiece, they were by far the most expensive part of the suspension aside from the shocks.  The weight of these bearings was a factor as well.  At 0.068 lbs. each, the four of them contributed a total of 0.272 lbs. to the buggy.

To get the necessary angular rocker travel, it was calculated that the gap in which the rocker was mounted would have to be on the order of 1.125” wide to accommodate a ¾” wide rocker.  Spacers would have to be placed between the bearing and the plates to reduce bending loads on the pins, but these spacers could not interfere with the motion of the rocker.  We manufactured the spacers ourselves out of aluminum with a taper on them, so they would have sufficient breadth to support the pin, but also stay clear of the motion of the rocker.

The other two pin sizes were decided based mostly on the standard available bushings and rod ends.  The bolt holding the shock-frame attach point would have to be ¼” because that was the only size spherical bearing that would fit in the shock.  This is what was used last year, and it held up fine (although last year they used slightly softer springs) Based on available bushings and rod ends, the pins for the shock-rocker and push-rod-rocker attach points were 3/8” and 5/16” respectively.  This would be more than strong enough.

    Mounting:
 

Mounting shocks and rockers to the frame required care as far as bringing the loads to nodes.  Since these mounts would take some of the largest loads on the buggy, the mounts would have to be beefy and therefore heavy.  The suspension mounting points were also likely to define the nodes and trussing on the buggy.  It was therefore in our interest to incorporate the suspension mounts with other elements on the buggy.  It has already been mentioned that the suspension cross-bar also serves to support the roller-clutch assembly.  This is a dramatic improvement over the heavy aluminum support system that was used last year.  We hoped to use this technique for the shock mounting points as well.

Because we wanted the push rods to be properly positioned to encounter loads from the front of the buggy, the suspension was one of the few things that would not be symmetric on the two buggy halves.  The shocks would always point forward from the rockers.  On the front, it was convenient to incorporate the suspension mounts with the shifter hub/crank support mounts.  Because of the high loads the mounts would encounter, we decided to build a 0.063” box structure covering the entire front corners of the buggy.  If we had not done this, the shock mounts would have been welded to the sides of the frame top tubes, with a minimum of a 1” lever arm.  This could easily have resulted in bending loads that could compromise the frame.  We anticipated potential loads as high as 1000lbs at the shock attach points. 

On the rear of the buggy, the shocks did not mount near the crank support mounts, the mounted midway down the frame top tubes.  Because of the bending loads described above, we were hesitant to mount the shocks without putting a crossbar across the frame at that point.  At first this seemed like a lot of unnecessary material, until we realized that the crossbar would double as a mount for our seat rail.  0.063” reinforcing gussets were welded between the frame top tubes and the seat crossbar to support the load of the shocks.  ( Early shock mount sketch )

In the end, all of our suspension/frame attach points were incorporated with other buggy systems, making trussing simpler and reducing the weight of the frame.

The outboard attachment points were done last, when the buggy was nearly assembled so we could visualize the location of the half shafts as the buggy moved through suspension and steering travel. (this was, unfortunately, quite beyond the range of our solid modeling capabilities)  On the back, it was easy to bring the mount almost to the tip of the A-arm.  We were actually hindered the most by the intrusion of the upright over the top of the bottom A-arm.  This is one area where a complete design beforehand could have been beneficial.  On the front, the steering motion of the Half shaft forced us to mount the push rod slightly more to the rear, but still close enough to the node that we were not concerned about the bending moment on the lower A-arm tube.  In order to alleviate all worries about this bending load, we put 0.040” plates spanning the tips of the lower A-arms.  The idea was to transfer some of the load to the other A-arm tube.  This also simplified the mounting of the push rod tabs, particularly on the rear where they could be closer to the centerline of the A-arm.  These tabs were made out of 0.063” sheet in order to withstand pullout and buckling loads.

Fabrication Notes:

    Rockers:
 

This year the fabrication of the rockers was much simpler than the method used last year.  With a lack of CNC programming experience present on this year’s team we decided we would be better off finding an alternative way manufacture the rockers.  Making a change much like the one made on the uprights we decided to move away from solid one-piece aluminum to a box style rocker made using steel plate .063” thick.  The three critical holes were drilled in the plate using a mill.  The final rocker design was drawn on paper and this paper was then attached to the plate.  The steel was then cut and ground until the piece matched the size of the paper design.  This process may not be the most accurate but the overall dimensions of the rocker are not critically important.  The most important part of the rocker design is the location of the 3 holes that attach to the pushrod, shock and frame.  As long as these holes are all in the correct place in relation to each other then it is ok if the rest of the steel is off by a few thousandths.

Once the plates were cut the bearing tubes were made out of 1” OD chro-moly.  This was the most difficult part of fabrication because in order for the bearings to be press fit into these holes, the tube had to be bored out to a tolerance of  0.9055" (+0, -0.0005).  Once the tubes were bored out and cut to length, the rocker was welded together.  The spherical bearings were then pressed into the tube.  The rockers were attached to the shock and pushrod using a series of pins, spacers, E-clips and bushings.  The E-clips were used instead of bolts because there was concern that bolts would be loosened by the constant rotation of the pin.  The thread of a bolt also weakens it significantly.  Pins with E-clips eliminated these problems.

     Inserts:
 

We used rod ends in several of the joints in our suspension.  They were at the outboard ends of our A-arms, and at both ends of our push-rods.  Inserts were made to fit the ID of the tubing, drilled and tapped for the appropriate rod ends.  The tubes were cut at an angle to provide a longer weld attaching the insert to the tube.  Inserts is an area in which some improvements could be made.  The inserts were made 1” long because we wanted to leave as little of the rod end exposed as possible, yet leave some room for adjustability.  Having too much of the threaded part of the rod-end exposed makes it a likely failure point.  The threads reduce the strength of the rod, having it extend very far from the insert can lead to bending loads.  We were most worried about this in the case of impulse bump loads on the A-arm rod-ends.  We therefore designed for leaving two threads exposed.  If it became absolutely necessary to move the rod end in further than two threads from the calculated location, we planned to cut down the insert.  For this reason, the inserts were all made 1” long.

An inch is absolutely unnecessary to carry the loads involved.  In most cases 4 or 5 threads would suffice.  It seems like the weight involved would be minimal, but there are 28 such inserts on the buggy, and it could be significant.

 Another note is that the rod ends on the outboard end of the push-rod are ¼-28 thread.  This is arguably insufficient for the thousand pound loads the push rods can experience, especially if a lot of the rod end is exposed to accommodate a heavier rider.  These rod ends were supposed to be 5/16-24 by design, but an ordering mistake left us with a size smaller.  This is a possible point of failure and should be changed on next year’s buggy.

Performance / Lessons Learned:

Overall the final design of the rockers performed exceptionally well.  They allowed for the inboard mounting of the suspension and smooth, progressive suspension travel. The final design was also strong enough and do not show any signs of wear or cracking.

We learned our most important lesson this year the first time we took the buggy out for a test run.  We had debated whether it was necessary to put a stiffening plate between the two parallel plates on the side of the rocker that was in compression, to prevent it from buckling.  Since we had not performed any calculations, we did not know whether this would be necessary.  We thought it was a good idea, but it was not high on our list of priorities.  The stiffening plate had not been welded on when we took the buggy out for its first test run and one of the rockers buckled.  We hastily welded on the plates, making the rocker much more rigid and allowing it to handle the loading.  The rockers simple construction allowed us to make a replacement for the buckled rocker in no time.  Once the plates were welded on the rockers did not fail again.  Such a failure during the race would have been costly, emphasizing the importance of finishing the buggy in time to test it before competition.

Our new rocker design performed up to all expectations.  The new rockers were made at a comparable weight to the ones on he old buggy but they do provide some distinct advantages.  The new design is much easier to manufacture, much easier to tweak, and also much cheaper.  There are some areas though that the rockers could still be improved.  Using the same style as on the old buggy it would have been impossible to save any more weight of the rockers because they could have failed if they were any thinner.  However, on the new design weight can definitely be saved.  A new design could use less material in order to decrease weight while still making rockers that are strong enough to handle the loading.  The thickness of the plates was determined by the pin-pullout loads.  This means that material could be removed from the rest of the rocker in order to save weight without losing performance.  Our rockers were of comparable weight to last years, but we feel our design would allow them to be much lighter.